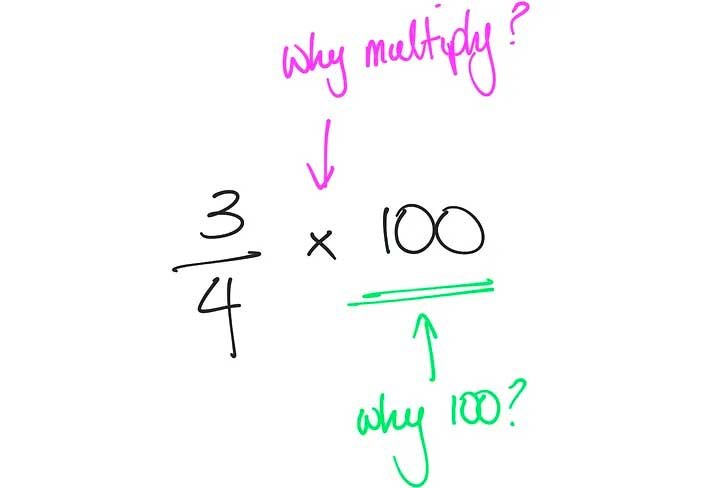A percentage is just a fraction you haven’t met yet!
I’m going to start by sharing a story. It's one of those stories that provides a sadly, not so uncommon look into our maths education system. It is a story about percentages gone terribly wrong! Let me set the scene...
I was happily shopping one afternoon, in a popular and well known beauty store in Melbourne's CBD. I've been known to dabble! After a particular set of products this day, I approached a lovely sales assistant who promptly set about explaining the products' ingredients and their various wondrous effects. The two items in question contained a substance called hyaluronic acid (HA). One, a 30 mL serum, contained 75% hyaluronic and the second, a 50 mL moisturiser, contained 30% hyaluronic. After covering most of the two products' various benefits, the sweet but seriously misinformed assistant proclaimed, "When used together, these products will provide your skin with over 100% Hyaluronic acid!" ...
...105% to be exact!
🤦🏻
Now, there are several problems with this statement, which I will try to highlight, before providing you with the correct solution to this problem. Before I do however, let's discuss percentages.
What is a percentage? Short answer, it is a fraction of a whole where the denominator, or the number of parts that the whole it partitioned into is 100. Let's look at a simple example.
Here we have the fraction 1/2 on the left and the fraction 50/100 on the right. Both represent the same value because the two fractions are what we call equivalent fractions. They are the same size even though the number of parts that each of the wholes or units are broken up into is different.
Here is another example. The fraction 1/4 is equivalent to 25/100.
As we continue with this method, we begin to notice that the numerator of any fraction with a denominator of 100 will represent our percentage. So 1/2 = 50%, 1/4 = 25%, 80/100 = 80% and so on. This is because the word percent comes from the Latin word per centum meaning 'hundred' or 'by the hundred'...so 50% means 50 parts of 100.
This may seem simple and obvious to many, but in my experience, percentages continue to remain problematic for a lot of people. One reason is that it is a concept that is taught very procedurally, with limited exposure to fractional models in the early stages of concept development. Think about how you teach, or were taught to convert fractions to percentages. For me, it was simple "Divide the top number by the bottom number and multiply that answer by 100". (PSA: For the love of everything that is good, please do not use this language!).
For now, let's follow our above method...
I'm not saying this is incorrect. It's a perfectly efficient way of making conversions with very little hassle. However, what it is not, is a method that builds any understanding of percentages nor provides students with the ability to access a problem should the scaffolds of the process fail. This method is students' final destination for percentages, not their starting point. Prior to using this method, we want them to understand why it works.
To do that, let us reverse engineer the problem.
We know that to convert 3/4 to a percentage, we divide the numerator by the denominator, and then multiply that answer by 100, so we will start with, why we multiply by 100.
When we are dealing with fraction multiplication, we want to change the language to make it conceptually easier to understand for students. (Have a read of my blog What the Fraction for full details of this method). Initial stages of multiplication refer to 'groups of', or just 'of' when performing a multiplication, so we're going to change our multiplication symbol to its literary symbol, 'of'.
When we make this simple change to language, it suddenly opens everything up. We now begin to make the connections to the earlier comparison models and are able to see why the calculator method works.
If we take it a step further, and look at the fraction 3/4 using a set or discrete model, it would look like this:
This idea of bringing any fraction back to the 100 is the fundamental principal of percentages. It is the how many parts "out of 100" principle, forever and always, because:
It is a lot easier to recognise the size of proportions when they are out of 100.
Our base-10 system uses hundredths in our representation of decimals. 75/100 = 0.75
Knowing what we now know, let's revisit the original statement: "When used together, these products will provide your skin with over 100% Hyaluronic acid!". We will now determine why it is incorrect and reveal the correct answer.
Let's begin by introducing the products in question. I rate them for those of you wondering.
The problem here lies in the fact that each of the products contains a different volume. One is 30 mL and the other is 50 mL and neither of these is 100 mL. We can simplify this by representing each of them as a 100 mL product and determining the HA content.
If there are 75 mL of HA in 100 mL of serum, and 30 mL of HA per 100 mL of moisturiser, how much will be in their respective container volumes? It's simple, just reduce the 100 mL to the quantity shown on each of the packagings, (30 mL of serum and 50 mL of moisturiser), and determine how much HA is now in those proportions.
So using these products together, does not deliver over 100% HA to our skin, as our delightful but misinformed sales assistant stated. Instead, we will end up with 37.5 mL of our total combined 80 mL of product. This equates to only 47% HA when the two products are used together, (I'll save this calculation for another time). Not bad, but still no where near 100%. I'd love to say that this revelation deterred me from paying the exorbitant price being asked for these products, but sadly it did not!
If you loved this post and would like to know more, why not reach out and book a chat or just email me at nadia@emmaths.com.au . I'd love to hear from you!